rightfrenzy.blogg.se

The general term for the given arithmetic sequence, using the common difference 𝑑 = 4 𝑎 + 4 𝑏 and the first term 𝑇 = 1 2 𝑎 + 9 𝑏 , is Let’s first calculate the difference between consecutive terms: The explicit formula for the 𝑛 t h term can be written in terms of the common difference and the first term, 𝑇 , as

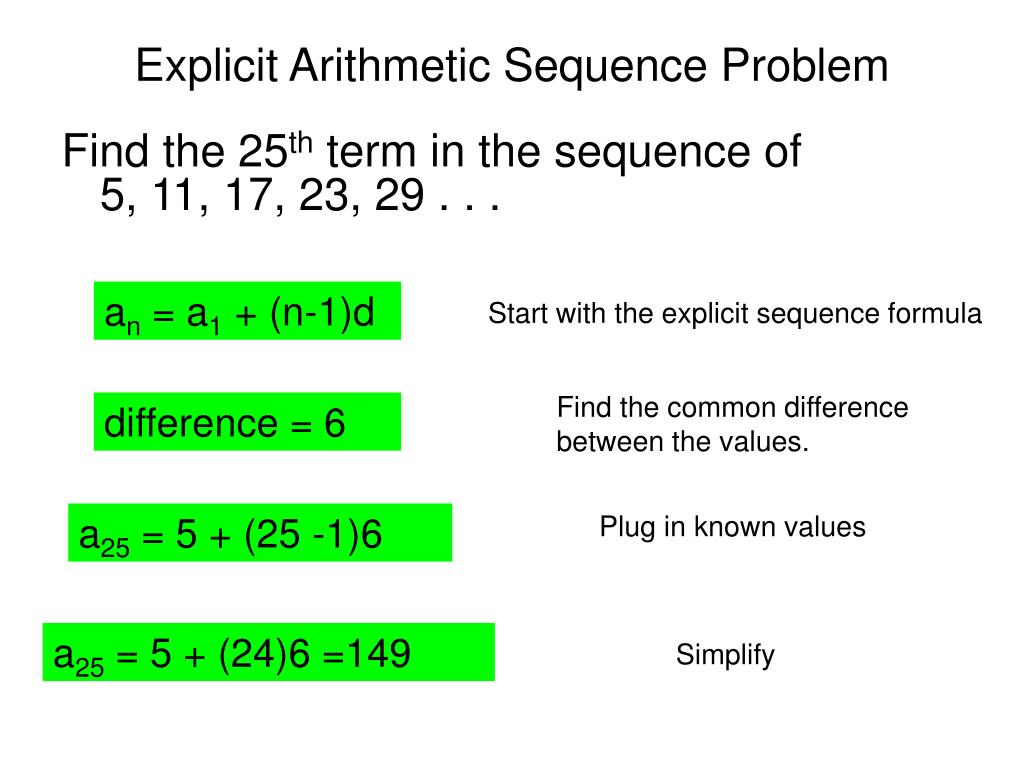
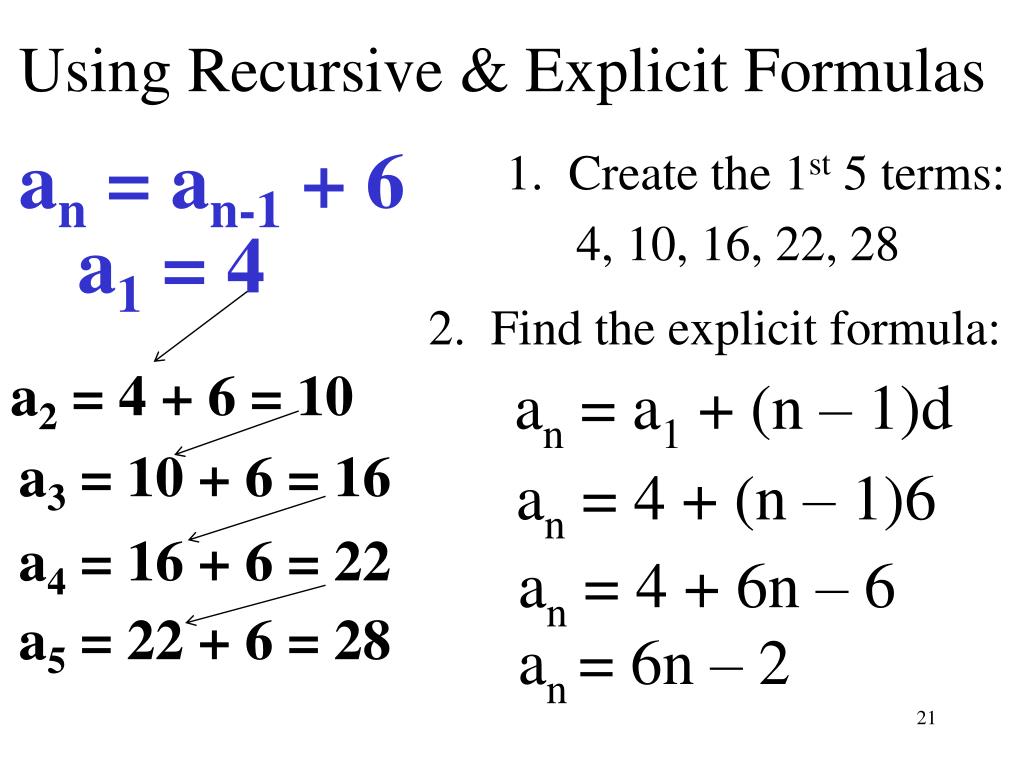
Recall that an arithmetic sequence is defined by a constant common difference, 𝑑, between any two consecutive terms. In this example, we want to find the value of a term in a given arithmetic sequence. Using the explicit formula, we will then determine the nineteenth term in the sequence.Įxample 4: Finding the Value of a Term in a Given Arithmetic Sequence In the next example, we will determine the explicit formula of an arithmetic sequence where the terms are expressed in terms of two parameters. įinally, we can determine the eighteenth term in the sequence by substituting 𝑛 = 1 8 to find The general term for the given arithmetic sequence, using the commonĭifference 𝑑 = 3 and first term 𝑇 = 4 , is Therefore, the first term of the sequence is 𝑇 = 4 . Since we know that 𝑇 = 1 9 , we can substitute this into the formula to obtain Now, we can determine the first term by substituting 𝑛 = 6 and 𝑑 = 3 into this formula: Recall that the explicit formula for the 𝑛 t h term of an arithmetic sequence can be written in terms of the common difference Therefore, this must be an arithmetic sequence with common difference 3. Recall that an arithmetic sequence is defined byĪ constant common difference, 𝑑, between any two consecutive terms.

We can see that each successive term can be obtained from the previous one by adding a common difference ( + 3). Now, let’s consider an example where we determine the general term from a table with values starting from the sixth term and then evaluate Hence, the general term of the sequence is 𝑇 = 2 𝑛 − 9 . The general term for the given arithmetic sequence, using the common difference 𝑑 = 2 and first term 𝑇 = − 7 , is Thus, we have a common difference 𝑑 = 2, which confirms that we have an arithmetic sequence. We are given the first few values of the sequence, 𝑇, 𝑇, 𝑇, 𝑇    . The explicit formulaįor the 𝑛 t h term can be written in terms of the common difference and the first term, 𝑇 , as In this example, we want to determine the general term of a given arithmetic sequence. No common difference so it is not an arithmetic sequence.Example 2: Finding the General Term of an Arithmetic Sequenceįind, in terms of 𝑛, the general term of the arithmetic sequence − 7, − 5, − 3, − 1, …. No common difference so it is not an arithmetic sequence.ģ.) 2, 5, 7, 12 in not because difference between first and second is 3, difference between second and third is 2, difference between third and fourth is 5. No common difference so it is not an arithmetic sequence.Ģ.) 1, 4, 9, 16 is not because difference between first and second is 3, difference between second and third is 5, difference between third and fourth is 7. The following are not examples of arithmetic sequences:ġ.) 2,4,8,16 is not because the difference between first and second term is 2, but the difference between second and third term is 4, and the difference between third and fourth term is 8. Here are some examples of arithmetic sequences:ġ.)7, 14, 21, 28 because Common difference is 7.Ģ.) 48, 45, 42, 39 because it has a common difference of - 3. An arithmetic sequence is a sequence (list of numbers) that has a common difference (a positive or negative constant) between the consecutive terms.